Class 8 - Mathematics
Cubes and Cube Roots - Exercise 7.2

Top Block 1
Question :1. Find the cube root of each of the following numbers by prime factorization method:
(i) 64
(ii) 512
(iii) 10648
(iv) 27000
(v) 15625
(vi) 13824
(vii) 110592
(viii) 46656
(ix) 175616
(x) 91125
(i) 64
(ii) 512
(iii) 10648
(iv) 27000
(v) 15625
(vi) 13824
(vii) 110592
(viii) 46656
(ix) 175616
(x) 91125
Answer :
(i) 64
Cuberoot(64) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2)
Cuberoot(64) = 2 x 2
= 4
(i) 64
Cuberoot(64) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2)
Cuberoot(64) = 2 x 2
= 4
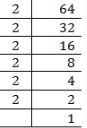
(ii) 512
Cuberoot(512) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2)
Cuberoot(512) = 2 x 2 x 2
= 8
Cuberoot(512) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2)
Cuberoot(512) = 2 x 2 x 2
= 8
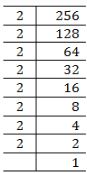
(iii) 10648
Cuberoot(10648) = Cuberoot(2 x 2 x 2 x 11 x 11 x 11)
Cuberoot(10648) = 2 x 11
= 22
Cuberoot(10648) = Cuberoot(2 x 2 x 2 x 11 x 11 x 11)
Cuberoot(10648) = 2 x 11
= 22

(iv) 27000
Cuberoot(27000) = Cuberoot(2 x 2 x 2 x 3 x 3 x 3 x 5 x 5 x 5)
Cuberoot(64) = 2 x 3 x 5
= 30
Cuberoot(27000) = Cuberoot(2 x 2 x 2 x 3 x 3 x 3 x 5 x 5 x 5)
Cuberoot(64) = 2 x 3 x 5
= 30

Mddle block 1
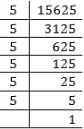
(v) 15625
Cuberoot(15625) = Cuberoot(5 x 5 x 5 x 5 x 5 x 5)
Cuberoot(15625) = 5 x 5
= 25
Cuberoot(15625) = Cuberoot(5 x 5 x 5 x 5 x 5 x 5)
Cuberoot(15625) = 5 x 5
= 25
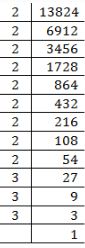
(vi) 13824
Cuberoot(13824) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 3 x 3 x 3)
Cuberoot(13824) = 2 x 2 x 2 x 3
= 24
Cuberoot(13824) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 3 x 3 x 3)
Cuberoot(13824) = 2 x 2 x 2 x 3
= 24
(vii) 110592
7 Cuberoot(110592) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 3 x 3 x 3)
Cuberoot(110592) = 2 x 2 x 2 x 2 x 3
= 48
7 Cuberoot(110592) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 3 x 3 x 3)
Cuberoot(110592) = 2 x 2 x 2 x 2 x 3
= 48

(viii) 46656
Cuberoot(46656) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 3 x 3 x 3 x 3 x 3 x 3)
Cuberoot(46656) = 2 x 2 x 3 x 3
= 36
Cuberoot(46656) = 2 x 2 x 3 x 3
= 36

(ix) 175616
Cuberoot(175616) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 7 x 7 x 7)
Cuberoot(175616) = 2 x 2 x 2 x 7
= 56
Cuberoot(175616) = Cuberoot(2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 7 x 7 x 7)
Cuberoot(175616) = 2 x 2 x 2 x 7
= 56

(x) 91125
Cuberoot(91125) = Cuberoot(3 x 3 x 3 x 3 x 3 x 3x 5 x 5 x 5)
Cuberoot(91125) = 3 x 3 x 5
= 45
Cuberoot(91125) = Cuberoot(3 x 3 x 3 x 3 x 3 x 3x 5 x 5 x 5)
Cuberoot(91125) = 3 x 3 x 5
= 45

Question :2. State true or false:
(i) Cube of any odd number is even.
(ii) A perfect cube does not end with two zeroes.
(iii) If square of a number ends with 5, then its cube ends with 25.
(iv) There is no perfect cube which ends with 8.
(v) The cube of a two digit number may be a three digit number.
(vi) The cube of a two digit number may have seven or more digits.
(vii) The cube of a single digit number may be a single digit number.
(i) Cube of any odd number is even.
(ii) A perfect cube does not end with two zeroes.
(iii) If square of a number ends with 5, then its cube ends with 25.
(iv) There is no perfect cube which ends with 8.
(v) The cube of a two digit number may be a three digit number.
(vi) The cube of a two digit number may have seven or more digits.
(vii) The cube of a single digit number may be a single digit number.
Answer :
(i) False
Since, 1³ = 1, 3³ = 27, 5³ = 125 …………… are all odd.
(ii) True
Since, a perfect cube ends with three zeroes. e.g. so on
10³ = 1000, 20³ = 8000, 30³ = 27000……. So on.
(iii) False
Since, 5² = 25, 5³ = 125, 15²= 225, 15³ = 3375
(Did not end with 25)
(iv) False
Since 12³ = 1728
[Ends with 8]
And 22³ = 10648
[Ends with 8]
(v) False Since10³ = 1000
[Four digit number]
And 11³ = 1331
[Four digit number]
(vi) False Since 99³ = 970299
[Six digit number]
(vii) True
1³ = 1
[Single digit number]
2³= 8
[Single digit number]
(i) False
Since, 1³ = 1, 3³ = 27, 5³ = 125 …………… are all odd.
(ii) True
Since, a perfect cube ends with three zeroes. e.g. so on
10³ = 1000, 20³ = 8000, 30³ = 27000……. So on.
(iii) False
Since, 5² = 25, 5³ = 125, 15²= 225, 15³ = 3375
(Did not end with 25)
(iv) False
Since 12³ = 1728
[Ends with 8]
And 22³ = 10648
[Ends with 8]
(v) False Since10³ = 1000
[Four digit number]
And 11³ = 1331
[Four digit number]
(vi) False Since 99³ = 970299
[Six digit number]
(vii) True
1³ = 1
[Single digit number]
2³= 8
[Single digit number]
Question :3. You are told that 1,331 is a perfect cube. Can you guess with factorization what is its cube root? Similarly guess the cube roots of 4913, 12167, 32768.
Answer :
We know that 10³
= 1000 and Possible cube of 11³= 1331
Since, cube of unit’s digit 1³ = 1
Therefore, cube root of 1331 is 11.
4913
We know that 7³ = 3437
Next number comes with 7 as unit place 17³= 4913
Hence, cube root of 4913 is 17.
12167
We know that 3³= 27
Here in cube, ones digit is 7
Now next number with 3 as ones digit
13³ = 2197
Andnext number with 3 as ones digit
23³ = 12167
Hence cube root of 12167 is 23.
32768
We know that 2³ = 8
Here in cube, ones digit is 8
Now next number with 2 as ones digit
12³= 1728
And next number with 2 as ones digit
22³= 10648
And next number with 2 as ones digit
32³ = 32768
Hence cube root of 32768 is 32.
We know that 10³
= 1000 and Possible cube of 11³= 1331
Since, cube of unit’s digit 1³ = 1
Therefore, cube root of 1331 is 11.
4913
We know that 7³ = 3437
Next number comes with 7 as unit place 17³= 4913
Hence, cube root of 4913 is 17.
12167
We know that 3³= 27
Here in cube, ones digit is 7
Now next number with 3 as ones digit
13³ = 2197
Andnext number with 3 as ones digit
23³ = 12167
Hence cube root of 12167 is 23.
32768
We know that 2³ = 8
Here in cube, ones digit is 8
Now next number with 2 as ones digit
12³= 1728
And next number with 2 as ones digit
22³= 10648
And next number with 2 as ones digit
32³ = 32768
Hence cube root of 32768 is 32.

