Class 8 - Mathematics
Understanding Quadrilaterals - Exercise 3.3

Top Block 1
Question: 1. Given a parallelogram ABCD. Complete each statement along with the definition or property used.

(ii) ∠DCB = ______________
(iii) OC = _____________
(iv) m∠DAB + m∠CDA = ________
Answer :
(i) AD = BC
[Since opposite sides of a parallelogram are equal]
(ii) ∠DCB = ∠DAB
[Since opposite angles of a parallelogram are equal]
(iii) OC = OA
[Since diagonals of a parallelogram bisect each other]
(iv) m∠DAB + m∠CDA = 180°
[Adjacent angles in a parallelogram are supplementary]
Question: 2. Consider the following parallelograms. Find the values of the unknowns x, y, z.
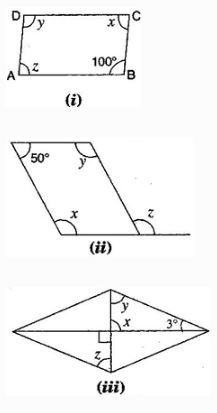
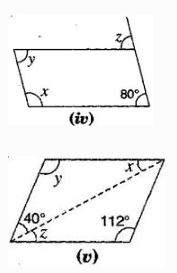
Answer :
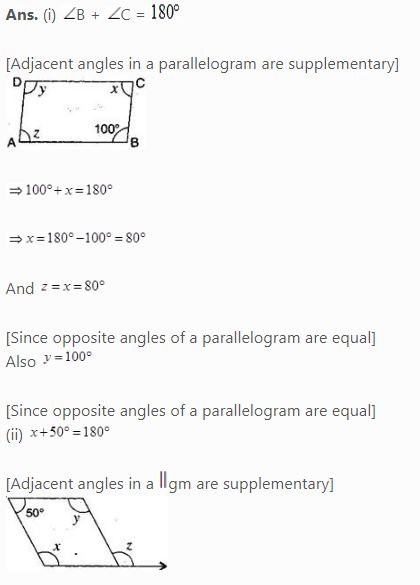
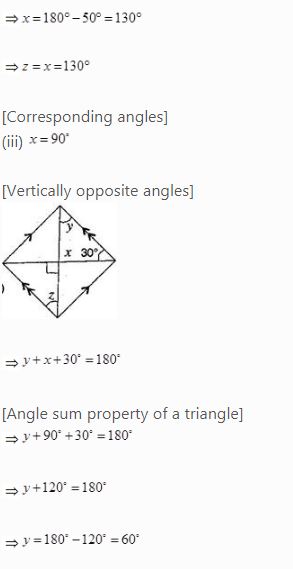
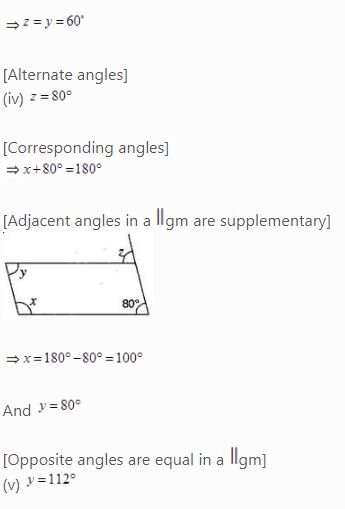
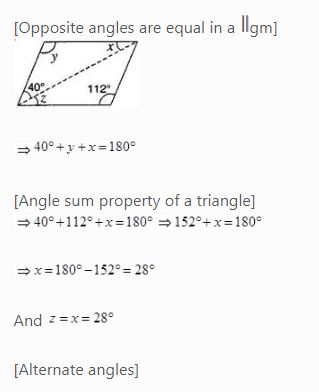
Mddle block 1
Question: 3. Can a quadrilateral ABCD be a parallelogram, if:
(i) ∠D + ∠B = 180°
(ii) AB = DC = 8 cm, AD = 4 cm and BC = 4.4 cm?
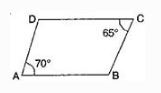
(iii) ∠A = and ∠C = 65°?
Answer :
(i) ∠D + ∠B = 180°
It can be, but here, it needs not to be.

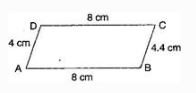
Since opposite angles are equal in parallelogram and here opposite angles are not equal in quadrilateral ABCD. Therefore it is not a parallelogram.
Question: 4. Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measures.
Answer :
ABCD is a quadrilateral in which angles ∠A = ∠C = 110°
Therefore, it could be a kite.

Question: 5. The measure of two adjacent angles of a parallelogram are in the ratio 3 : 2. Find the measure of each of the angles of the parallelogram.
Answer :
Let two adjacent angles be and
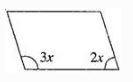
∴ 3𝓍 + 2𝓍 = 180°
⇒ 5𝓍 = 180°
⇒ 𝓍 = 180°⁄5
⇒ 𝓍 = 36°
∴ One Angle = 3𝓍 = 3 x 36° = 108°
And Another angle = 2𝓍 = 2 x 36° = 72°
Question: 6. Two adjacent angles of a parallelogram have equal measure. Find the measure of the angles of the parallelogram.
Answer :
Let each adjacent angle be 𝓍
Since the adjacent angles in a parallelogram are supplementary.
∴ 𝓍 + 𝓍 = 180°
⇒ 2𝓍 = 180°
⇒ 𝓍 = 180°⁄2
⇒ 𝓍 = 90°
Hence, each adjacent angle is 90°.
Question: 7. The adjacent figure HOPW is a parallelogram. Find the angle measures 𝓍, 𝓎 and 𝓏 State the properties you use to find them.

Answer :

Question: 8. The following figures GUNS and RUNS are parallelograms. Find 𝓍 and 𝓎. (Lengths are in cm)

Answer :


Question: 9. In the figure, both RISK and CLUE are parallelograms. Find the value of 𝓍.
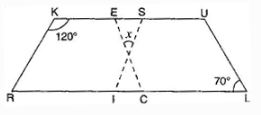
Answer :
Question: 10. Explain how this figure is a trapezium. Which is its two sides are parallel?
Answer :

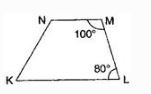
Question: 11. Find m∠C in figure , if AB∥CD

Answer :

Question: 12. Find the measure of ∠P and ∠S if SP∥RQin given figure. (If you find m∠R is there more than one method to find m∠P)

Answer :



