Class 8 - Mathematics
Visualising Solid Shapes - Exercise 10.2rm

Top Block 1
Question :1.Can a polyhedron have for its faces
Answer :
(i) 3 triangles?
(ii) 4 triangles?
(iii) a square and four triangles?
A polyhedron is bounded by four or more than four polygonal faces.
(i) No, it is not possible that a polyhedron has 3 triangles for its faces.
(ii) Yes, 4 triangles can be the faces of a polyhedron.
(iii) Yes, a square and 4 triangles can be the faces of a polyhedron.
(i) 3 triangles?
(ii) 4 triangles?
(iii) a square and four triangles?
A polyhedron is bounded by four or more than four polygonal faces.
(i) No, it is not possible that a polyhedron has 3 triangles for its faces.
(ii) Yes, 4 triangles can be the faces of a polyhedron.
(iii) Yes, a square and 4 triangles can be the faces of a polyhedron.
Question :2.Is it possible to have a polyhedron with any given number of faces?
Hint: Think of a pyramid.
Hint: Think of a pyramid.
Answer :
Yes, it can be possible only
if the number of faces is four or more than four.
Yes, it can be possible only
if the number of faces is four or more than four.
Question :3. Which are prisms among the following?
Answer :
Since, a prism is a polyhedron having two of its faces congruent and parallel, where as other faces
are parallelogram.
(i) No, a nail is not a prism.
(ii) Yes, unsharpened pencil is a prism.
(iii) No, table weight is not a prism.
(iv) Yes, box is a prism.
Since, a prism is a polyhedron having two of its faces congruent and parallel, where as other faces
are parallelogram.
(i) No, a nail is not a prism.
(ii) Yes, unsharpened pencil is a prism.
(iii) No, table weight is not a prism.
(iv) Yes, box is a prism.
Question :4. (i) How are prisms and cylinders alike?
(ii) How are pyramids and cones alike?
(ii) How are pyramids and cones alike?
Answer :
(i) Both of the prisms and cylinders have their base and top as congruent faces and parallel
to each other. Also, a prism becomes a cylinder as the number
of sides of its base becomes larger and larger.
(ii) The pyramid and cones are alike becomes their lateral faces meet at a vertex.
Also a pyramid becomes a cone as the number of sides of its base becomes larger and larger.
Question :5. Is a square prism same as a cube? Explain.
Answer :
No, not always, because it can be a cuboid also.
No, not always, because it can be a cuboid also.
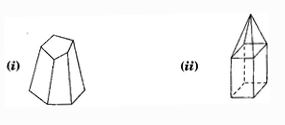
Question :6.Verify Euler’s formula for these solids.
Mddle block 1
Answer :
(i) In figure (i), we have
⇒ F = 7, V = 10 and E = 15
∴F + V = 7 + 10 = 17
⇒ F + V – E = 17 – 15 = 2
i.e. F + V – E = 2
Thus, Euler’s formula is verified.
(ii) In figure (ii), we have
⇒ F = 9, V = 9 and E = 16
⇒ F + V = 9 + 9 = 18
and F + V – E = 18 – 16 = 2
i.e. F + V – E = 2
Thus, Euler’s formula is verified.
(i) In figure (i), we have
⇒ F = 7, V = 10 and E = 15
∴F + V = 7 + 10 = 17
⇒ F + V – E = 17 – 15 = 2
i.e. F + V – E = 2
Thus, Euler’s formula is verified.
(ii) In figure (ii), we have
⇒ F = 9, V = 9 and E = 16
⇒ F + V = 9 + 9 = 18
and F + V – E = 18 – 16 = 2
i.e. F + V – E = 2
Thus, Euler’s formula is verified.
Question :7.Using Euler’s formula find the unknown.
| Faces | ? | 5 | 20 |
| Vertices | 6 | ? | 12 |
| Edges | 12 | 9 | ? |
Answer :
(i) Here, V = 6 and E = 12
Since F + V – E = 2
∴F + 6 – 12 = 2
⇒ or F – 6 = 2
⇒ or F = 2 + 6 = 8
(ii) Here, F = 5 and E = 9
Since F + V – E = 2
⇒ or 5 + V – 9 = 2
⇒ or V – 4 = 2
⇒ or v = 2 + 4 = 6
(iii) Here F = 20 V = 12
Since, F + V – E = 2
∴20 + 12 – E = 2
⇒ or 20 + 12 – E = 2
⇒ 32 – E = 2
⇒ or E = 32 – 2 = 30
(i) Here, V = 6 and E = 12
Since F + V – E = 2
∴F + 6 – 12 = 2
⇒ or F – 6 = 2
⇒ or F = 2 + 6 = 8
(ii) Here, F = 5 and E = 9
Since F + V – E = 2
⇒ or 5 + V – 9 = 2
⇒ or V – 4 = 2
⇒ or v = 2 + 4 = 6
(iii) Here F = 20 V = 12
Since, F + V – E = 2
∴20 + 12 – E = 2
⇒ or 20 + 12 – E = 2
⇒ 32 – E = 2
⇒ or E = 32 – 2 = 30
Question :8.Can polyhedron have 10 faces, 20 edges and 15 vertices?
Answer :
Here, F = 10, E = 20 and V = 15
We have:
⇒ F + V – E = 2
⇒ 10 + 15 – 20 = 2
⇒ or 25 – 20 = 2
⇒ or 5 = 2 which is not true
i.e. F + V – E 2
Thus, such a polyhedron is not possible.
Here, F = 10, E = 20 and V = 15
We have:
⇒ F + V – E = 2
⇒ 10 + 15 – 20 = 2
⇒ or 25 – 20 = 2
⇒ or 5 = 2 which is not true
i.e. F + V – E 2
Thus, such a polyhedron is not possible.

