Class 10 - Mathematics
Polynomials - Exercise 2.4

Top Block 1
Exercise 2.4
Question : 1:Verify that the numbers given alongside of the cubic polynomials below are their zeroes.
Also verify the relationship between the zeroes and the coefficients in each case:
(i) 2x3 + x2 – 5x + 2; 1/2, 1, -2 (ii) x3 – 4x2 + 5x – 2; 2, 1, 1
Answer :
(i) Let p(x) = 2x3 + x2 – 5x + 2
Now, p(1/2) = 2(1/2)3 + (1/2)2 – 5(1/2) + 2
= 2 * 1/8 + 1/4 – 5/2 + 2
= 1/4 + 1/4 – 5/2 + 2
= 1/2 + 2 – 5/2
= 5/2 – 5/2
= 0
So, 1/2 is one of the zero of p(x).
p(1) = 2(1)3 + (1)2 – 5(1) + 2
= 2 + 1 – 5 + 2
= 5 – 5
= 0
So, 1 is one of the zero of p(x).
p(-2) = 2(-2)3 + (-2)2 – 5(-2) + 2
= -2 * 8 + 4 + 10 + 2
= -16 + 16
= 0
So, -2 is one of the zero of p(x).
Therefore, 1/2, 1 and -2 are the zeroes of p(x).
Let a = 1/2, b = 1, c = -2
Now,
a + b + c = 1/2 + 1 + (-2) = 1/2 + 1 -2 = -1/2 = -(1)/2 = -(coefficient of x2)/( coefficient of x3)
ab + bc + ca = 1/2 * 1 + 1 * (-2) + (-2) * 1/2
= 1/2 – 2 – 1
= 1/2 – 3
= -5/2
= -(coefficient of x)/( coefficient of x3)
abc = 1/2 * 1 * (-2) = -1 = -2/2 = -(constant term)/( coefficient of x3)
Hence, the relation between zeroes and coefficients is verified.
(ii) Let p(x) = x3 – 4x2 + 5x – 2
Now, p(2) = 23 – 4 * 22 + 5 * 2 – 2
= 8 – 16 + 10 – 2
= 18 – 18
= 0
So, 2 is one of the zero of p(x).
p(1) = 13 – 4(1)2 + 5(1) – 2
= 1 – 4 + 5 + 2
= 6 – 6
= 0
So, 1 is one of the zero of p(x).
Therefore, 2, 1 and 1 are the zeroes of p(x).
Let a = 2, b = 1, c = 1
Now,
a + b + c = 2 + 1 + 1 = 4 = -(-4)/1 = -(coefficient of x2)/( coefficient of x3)
ab + bc + ca = 2 * 1 + 1 * 1 + 1 * 2
= 2 + 1 + 2
= 5
= 5/1
= coefficient of x/coefficient of x3
abc = 2 * 1 * 1 = 2 = -(-2)/1 = -(constant term)/( coefficient of x3)
Hence, the relation between zeroes and coefficients is verified.
Question : 2:Find a cubic polynomial with the sum, sum of the product of its zeroes taken two at a time, and the product of its zeroes as 2, -7, -14 respectively.
Answer :
Let p(x) = ax3 + bx2 + cx + d be a cubic polynomial whose zeroes are α, β and γ.
Given that, α + β + γ = 2
αβ + βγ + αγ = -7
αβγ = -14
We know that
α + β + γ = -(coefficient of x2)/( coefficient of x3)
αβ + βγ + αγ = coefficient of x/coefficient of x3
αβγ = -(constant term)/( coefficient of x3)
Therefore,
α + β + γ = -(coefficient of x2)/( coefficient of x3) = -b/a = 2/1
αβ + βγ + αγ = coefficient of x/coefficient of x3 = c/a = -7/1
αβγ = -(constant term)/( coefficient of x3) = -d/a = -14/1
On comparing, we get
a = 1, b = -2, c = -7 and d = 14
Hence, the required cubic polynomial is p(x) = x3 – 2x2 – 7x + 14
Question : 3:If the zeroes of the polynomial x3 – 3x2 + x + 1 are a – b, a, a + b, find a and b.
Answer :
We know that
Sum of zeroes = -(coefficient of x2)/( coefficient of x3)
⇒ a – b + a + a + b = -(-3)/1
⇒ 3a = 3
⇒ a = 3/3
⇒ a = 1
Product of zeroes = -(constant term)/( coefficient of x3)
⇒ (a – b)(a)(a + b) = -1/1
⇒ (1 – b)(1)(1 + b) = -1 [By putting value of a]
⇒ 1 – b2 = -1
⇒ b = 1 + 1
⇒ b2 = 2
⇒ b = ±√2
Hence, a = 1 and b = ±√2
Question : 4:If two zeroes of the polynomial x4 – 6x3 – 26x2 + 138x – 35 are 2 ± √3, find other zeroes.
Answer :
Let p(x) = x4 – 6x3 – 26x2 + 138x – 35
Given that 2 + √3 and 2 – √3 are two of the zeroes of p(x)
So, (x – 2 – √3) and (x – 2 + √3) are the factors of p(x)
Therefore, (x – 2)2 – (√3)2 is a factor of p(x)
⇒ x2 – 2x + 4 – 3 is a factor of p(x)
⇒ x2 – 2x + 1 is a factor of p(x)
Now, divide x4 – 6x3 – 26x2 + 138x – 35 by x2 – 2x + 1, we get
Mddle block 1
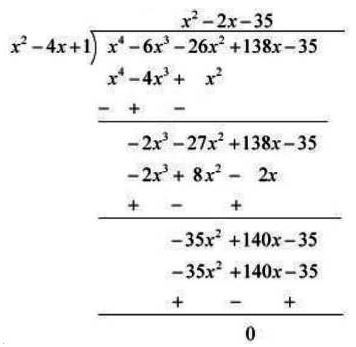
Therefore,
p(x) = x4 – 6x3 – 26x2 + 138x – 35
= (x2 – 2x + 1)( x2 – 2x – 35)
= (x2 – 2x + 1)( x2 – 7x + 5x – 35)
= (x2 – 2x + 1){x(x – 7) + 5(x – 7)}
= (x2 – 2x + 1)(x – 7)(x + 5)
To get the other zeroes, put x + 5 = 0 and x – 7 = 0
⇒ x = -5, 7
Hence, the other two zeroes are -5 and 7
Question : 5:If the polynomial x4 – 6x3 + 16x2 – 25x + 10 is divided by another polynomial x2 – 2x + k, the remainder comes out to be x + a, find k and a.
Answer :
Given that
Divisor = x2 – 2x + k
Dividend = x4 – 6x3 + 16x2 – 25x + 10
Remainder = x + a
We know that
Dividend = Divisor * Quotient + Remainder
⇒ x4 – 6x3 + 16x2 – 25x + 10 = (x2 – 2x + k) * Quotient + (x + a)
⇒ x4 – 6x3 + 16x2 – 25x + 10 – x – a = (x2 – 2x + k) * Quotient
⇒ x4 – 6x3 + 16x2 – 26x + 10 – a = (x2 – 2x + k) * Quotient
⇒ Quotient = (x4 – 6x3 + 16x2 – 26x + 10 – a)/ (x2 – 2x + k)
So, if the polynomial x4 – 6x3 + 16x2 – 26x + 10 – a is divisible by x2 – 2x + k, the remainder will
be zero.
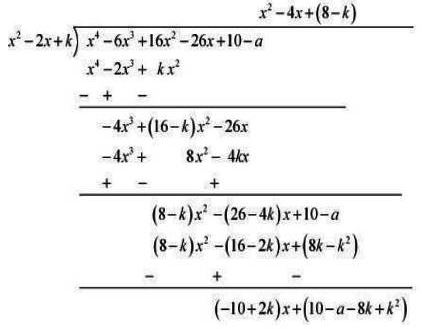
On comparing,
-10 + 2k = 0
⇒ 2k = 10
⇒ k = 10/2
⇒ k = 5
and 10 – a – 8k + k2 = 0
⇒ 10 – a – 8 * 5 + 52 = 0
⇒ 10 – a – 40 + 25 = 0
⇒ 35 – a – 40 = 0
⇒ -5 – a = 0
⇒ a = -5
Hence, k = 5 and a = -5

