Class 6 - Mathematics : Practical Geometry
Exercise : 14.6

Top Block 1
NCERT Solutions Class 6 Mathematics Practical Geometry Ex 14.6
Question: 1.Draw ∠POQ of measure 75o and find its line of symmetry.
Answer :
Steps of construction:

(b) Place the pointer of the compasses at O and draw an arc of any radius which intersects the line l at A.
(c) Taking same radius, with centre A, cut the previous arc at B.
(d) Join OB, then ∠BOA = 60oo.
(e) Taking same radius, with centre B, cut the previous arc at C.
(f) Draw bisector of ∠BOC. The angle is of 90o. Mark it at D. Thus, ∠DOA = 90o
(g) Draw OP as bisector of ∠DOB.
Thus, ∠POA = 75o
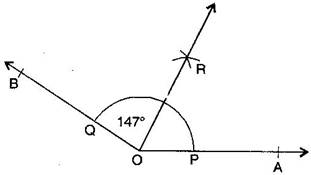
Question: 2.Draw an angle of measure 147o and construct its bisector.
Answer :
Steps of construction:
Mddle block 1
(a) Draw a ray![]()
(b) With the help of protractor, construct ∠AOB = 147o.
(c) Taking centre O and any convenient radius, draw an arc which intersects the arms OA and OB at P and Q respectively.
(d) Taking P as centre and radius more than half of PQ, draw an arc.
(e) Taking Q as centre and with the same radius, draw another arc which intersects the previous at R.
(f) Join OR and produce it.
Thus, OR is the required bisector of ∠AOB.
Question: 3.Draw a right angle and construct its bisector.
Answer :
Steps of construction:

(b) Taking O as centre and convenient radius, draw an arc which intersects PQ at A and B.
(c) Taking A and B as centers and radius more than half of AB, draw two arcs which intersect each other at C.
(d) Join OC. Thus, ∠COQ is the required right angle.
(e) Taking B and E as centre and radius more than half of BE, draw two arcs which intersect each other at the point D.
(f) Join OD. Thus, OD is the required bisector of ∠COQ.
Question: 4. Draw an angle of measure 153o and divide it into four equal parts.
Answer :
Steps of construction:
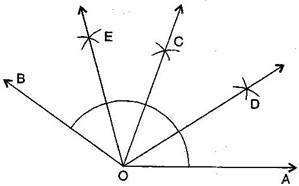
(a) Draw a ray ![]()
(b) At O, with the help of a protractor, construct ∠AOB = 153o.
(c) Draw OC as the bisector of ∠AOB.
(d) Again, draw OD as bisector of ∠AOC.
(e) Again, draw OE as bisector of ∠BOC.
(f) Thus, OC, OD and OE divide ∠AOB in four equal arts.
Question: 5.Construct with ruler and compasses, angles of following measures:
(a) 60o
(b) 30o
(c) 90o
(d) 120o
(e) 45o
(f) 135o
Answer :
Steps of construction:
(a) 60o
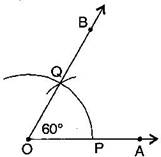
(i) Draw a ray 
(ii) Taking O as centre and convenient radius, mark an arc, which intersects  at P.
at P.
(iii) Taking P as centre and same radius, cut previous arc at Q.
(iv) Join OQ.
Thus, ∠BOA is required angle of 60o.
(b) 30o

(i) Draw a ray 
(ii) Taking O as centre and convenient radius, mark an arc, which intersects  at P.
at P.
(iv) Join OQ. Thus, ∠BOA is required angle of 60o.
(v) Put the pointer on P and mark an arc.
(vi) Put the pointer on Q and with same radius, cut the previous arc at C.
Thus, ∠COA is required angle of 30o.
(c)90o
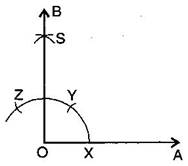
(i) Draw a ray 
(ii) Taking O as centre and convenient radius, mark an arc, which intersects  at X.
at X.
(iii) Taking X as centre and same radius, cut previous arc at Y.
(iv) Taking Y as centre and same radius, draw another arc intersecting the same arc at Z.
(v) Taking Y and Z as centers and same radius, draw two arcs intersecting each other at S.
(vi) Join OS and produce it to form a ray OB.
Thus, ∠BOA is required angle of 90o.
(d)120o
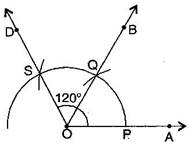
(i) Draw a ray 
(ii) Taking O as centre and convenient radius, mark an arc, which intersects  at P.
at P.
(iii) Taking P as centre and same radius, cut previous arc at Q.
(iv) Taking Q as centre and same radius cut the arc at S.
(v) Join OS.
Thus, ∠AOD is required angle of 120o.
(e)45o
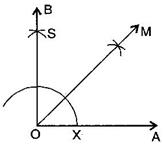
(i) Draw a ray 
(ii) Taking O as centre and convenient radius, mark an arc, which intersects  at X.
at X.
(iii) Taking X as centre and same radius, cut previous arc at Y.
(iv) Taking Y as centre and same radius, draw another arc intersecting the same arc at Z.
(v) Taking Y and Z as centers and same radius, draw two arcs intersecting each other at S.
(vi) Join OS and produce it to form a ray OB. Thus, ∠BOA is required angle of 90o
(vii) Draw the bisector of ∠BOA.
Thus, ∠MOA is required angle of 45o
(f)135o

(i) Draw a line PQ and take a point O on it.
(ii) Taking O as centre and convenient radius, mark an arc, which intersects PQ at A and B.
(iii) Taking A and B as centers and radius more than half of AB, draw two arcs intersecting each other at R.
(iv) Join OR. Thus, ∠QOR = ∠POQ = 90o
(v) Draw 
thus, ∠QOD is required angle of 135o
Question: 6.Draw an angle of measure 45o and bisect it.
Answer :
Steps of construction:

(a)Draw a line PQ and take a point O on it.
(b)Taking O as centre and a convenient radius, draw an arc which intersects PQ at two points A and B.
(c)Taking A and B as centers and radius more than half of AB, draw two arcs which intersect each other at C.
(d)Join OC. Then ∠COQ is an angle of 90o
(e)Draw 
as the bisector of ∠COE. Thus, ∠QOE = 45o . Again Draw 

Question: 7.Draw an angle of measure 135o and bisect it.
Answer :
Steps of construction:

(a) Draw a line PQ and take a point O on it.
(b) Taking O as centre and convenient radius, mark an arc, which intersects PQ at A and B.
(c) Taking A and B as centers and radius more than half of AB, draw two arcs intersecting each other at R.
(d) Join OR. Thus, ∠QOR = ∠POQ = 90o
(e) Draw  the bisector of ∠POR. Thus, ∠QOD is required angle of 135o
the bisector of ∠POR. Thus, ∠QOD is required angle of 135o
(f) Now, draw  as the bisector of ∠QOD.
as the bisector of ∠QOD.
Thus, ∠QOE = ∠DOE = 671⁄2o
Question: 8.Draw an angle of 70o. Make a copy of it using only a straight edge and compasses.
Answer :
Steps of construction:

(a) Draw an angle 70o with protractor, i.e., ∠POQ=70o
(b) Draw a ray 
(c) Place the compasses at O and draw an arc to cut the rays of ∠POQ at L and M.
(d) Use the same compasses, setting to draw an arc with A as centre, cutting AB at X.
(e) Set your compasses setting to the length LM with the same radius.
(f) Place the compasses pointer at X and draw the arc to cut the arc drawn earlier at Y.
(g) Join AY.
Thus, ∠YAX=70o
Question: 9.Draw an angle of 40o. Copy its supplementary angle.
Answer :
Steps of construction:
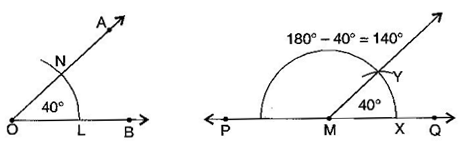
(b) Draw a line PQ.
(c) Take any point M on PQ.
(d) Place the compasses at O and draw an arc to cut the rays of ∠AOB at L and N.
(e) Use the same compasses setting to draw an arc O as centre, cutting MQ at X.
(f) Set your compasses to length LN with the same radius.
(g) Place the compasses at X and draw the arc to cut the arc drawn earlier Y.
(h) Join MY.
Thus, ∠QMY = 40o and ∠PMY is supplementary of it.

