Class 8 - Mathematics
Algebraic Expressions and Identities - Exercise 11.3

Top Block 1
Question :1. There are two cuboidal boxes as shown in the adjoining figure. Which box requires the lesser amount of material to make?

Answer :
(a) Given: Length of cuboidal box 𝓁 = 60 cm
Breadth of cuboidal box 𝒷 = 40 cm
Height of cuboidal box 𝒽 = 50 cm
∴ Total surface area of cuboidal box
= 2(𝓁𝒷 + &bscrcm;𝒽 + 𝒽𝓁)
= 2 (60 x 40 + 40 x 50 + 50 x 60)
= 2 (2400 + 2000 + 3000)
= 2 x 7400 = 14800 ²
(b) Given: Length of cuboidal box 𝓁 = 50 cm
Breadth of cuboidal box 𝒷 = 50 cm
Height of cuboidal box 𝒽 = 50 cm
∴ Total surface area of cuboidal box
= 2(𝓁𝒷 + &bscrcm;𝒽 + 𝒽𝓁)
= 2 (50 x 50 + 50 x 50 + 50 x 50)
= 2 (2500 + 2500 + 2500)
= 2 x 7500 = 15000 cm²
Hence cuboidal box 𝒶 requires the lesser amount of materal to make, since surface area of box 𝒶 is less than that of box 𝒷.
Question :2. A suitcase with measures 80 cm x 48 cm x 24 cm is to be covered with a tarpaulin cloth. How many meters of tarpaulin of width 96 cm is required to cover 100 such suitcases?
Answer :
Given: Length of suitcase box 𝓁 = 80 cm, Breadth of suitcase box 𝒷 = 48 cm
And Height of cuboidal box 𝒽 = 24 cm
∴ Total surface area of suitcase box
= 2(𝓁𝒷 + &bscrcm;𝒽 + 𝒽𝓁)
= 2 (80 x 48 + 48 x 24 + 24 x 80)
= 2 (3840 + 1152 + 1920)
= 2 x 6912 = 13824 cm²
Area of Tarpaulin cloth = Surface area of suitcase
𝓁 x 𝒷 = 13824
𝓁 x 96 = 13824 x 96 = 144 cm
Required tarpaulin for 100 suitcases
= 144 100 = 14400 cm = 144 m
Hence tarpaulin cloth required to cover 100 suitcases is 144 m.
Question :3. Find the side of a cube whose surface area id 600 cm².
Answer :
Here Surface area of cube = 600 cm²
6𝓁² = 600
𝓁² = 100
𝓁 = 10 cm
Hence the side of cube is 10 cm
Question :4. Rukshar painted the outside of the cabinet of measure 1 m x 2 m x 1.5 m. How much surface area did she cover if she painted all except the bottom of the cabinet?

Mddle block 1
Answer :
Here, Length of cabinet 𝓁 = 2 m, Breadth of cabinet 𝒷 = 1 m
And Height of cabinet 𝒽 = 1.5 m
∴ Surface area of cabinet = 𝓁𝒷 +2(𝒷𝒽 + 𝒽𝓁)
= 2 x 1 + 2 (1 x 1.5 + 1.5 x 2)
= 2 + 2 (1.5 + 3.0)
= 2 + 9.0
= 11 m²
Hence required surface area of cabinet is 11 m².
Question :5. Daniel is paining the walls and ceiling of a cuboidal hall with length, breadth and height of 15 m, 10 m and 7 m respectively. From each can of paint 100m² of area is painted. How many cans of paint will she need to paint the room?
Answer :
Here, Length of wall 𝓁 = 15 m, Breadth of wall 𝒷 = 10 m
And Height of wall 𝒽 = 7 m
∴ Total Surface area of classroom
= 𝓁𝒷 +2(𝒷𝒽 + 𝒽𝓁)
= 15 x 10 + 2 (10 x 7 + 7 x 15)
= 150 + 2 (70 + 105)
= 150 + 350
= 500m²
Now Required number of cans
= Area of Hall⁄Area of one can = 500⁄100 = 5 cans
Hence 5 cans are required to paint the room.
Question :6. Describe how the two figures below are alike and how they are different. Which box has larger lateral surface area?
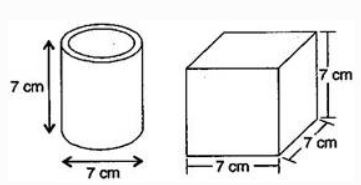
Answer :
Given: Diameter of cylinder = 7 cm
∴ Radius of cylinder r = 7⁄2 cm
And Height of cylinder 𝒽 = 7 cm
Lateral surface area of cylinder = 2πr𝒽
= 2 x 3.14 x 7⁄2 x 7
= 154 cm²
Now lateral surface area of cube
= 4𝓁² = 4 x 7² = 4 x 49 = 196 cm²
Hence the cube has larger lateral surface area.
Question :7. A closed cylindrical tank of radius 7 m and height 3 m is made from a sheet of metal. How much sheet of metal is required?
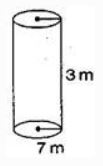
Answer :
Given: Radius of cylindrical tank r = 7 m
Height of cylindrical tank 𝒽 = 3 m
Total surface area of cylindrical tank
= 2πr(𝒽 + r)
= 2 x 3.14 x 7(3 + 7)
= 44 x 10 = 440 m²
Hence 440 m² metal sheet is required.
Question :8. The lateral surface area of a hollow cylinder is 4224 cm². It is cut along its height and formed a rectangular sheet of width 33 cm. Find the perimeter of rectangular sheet?
Answer :
Given: Lateral surface area of hollow cylinder = 4224 cm²
And Height of hollow cylinder = 33 cm
Curved surface area of hollow cylinder = 2πr𝒽
⇒ 4224 = 2 x 3.14 x r x 33
r = (4224)⁄(2 x 33) = 64⁄3.14 cm
Now Length of rectangular sheet = 2πr.
𝓁 = 2 x 3.14 x 64⁄3.14 = 128 cm
Perimeter of rectangular sheet = 2(𝓁 + 𝒷)
= 2(128 + 33) = 2 x 161 = 322 cm
Hence perimeter of rectangular sheet is 322 cm.
Question :9. A road roller takes 750 complete revolutions to move once over to level a road. Find the area of the road if the diameter of a road roller is 84 cm and length 1 m.
Answer :
Given: Diameter of road roller = 84 cm
∴ Radius of road roller r = 𝒹⁄2 = 84⁄2
= 42 cm
Length of road roller 𝓁 = 1 m = 100 cm
Curved surface area of road roller = 2πr𝒽 = 2 x 3.14 x 42 x 100 = 26400 cm²2
∴ Area covered by road roller in 750 revolutions = 26400 x 750
= 1,98,00,000 cm²
= 1980 m2 [∵1 m² = 10,000 cm²]
Hence the area of the road is 1980m².
Question :10. A company packages its milk powder in cylindrical container whose base has a diameter of 14 cm and height 20 cm. Company places a label around the surface of the container (as shown in figure). If the label is placed 2 cm from top and bottom, what is the area of the label?

Answer :
Given: Diameter of cylindrical container = 14 cm
∴ Radius of cylindrical container r = 𝒹⁄2 = 14⁄2 = 7 cm
Height of cylindrical container = 20 cm
Height of the label 𝒽 = 20 – 2 – 2
= 16 cm
Curved surface area of label = 2πr𝒽
= 2 x 3.14 x 7 x 16 = 704 cm²
Hence the area of the label of 704 cm².

