Class 9 - Mathematics
Circles - Exercise 10.3

Top Block 1
Exercise 10.3
Question : 1 : Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
Answer :
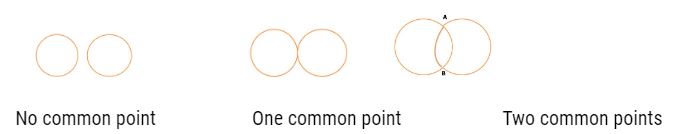
No common point One common point Two common points
Hence, the maximum number of common point is two.
Question : 2: Suppose you are given a circle. Give a construction to find its centre.
Answer :
Steps of Construction:
- Take arc PQ of the given circle.
- Take a point R on the arc PQ and draw chords PR and RQ.
- Draw perpendicular bisectors of PR and RQ. These
perpendicular bisectors intersect at point O.
Hence, point O is the centre of the given circle.

Mddle block 1
Question : 3: If two circles intersect at two points, prove that their centres lie on the perpendicular bisector of the common chord.
Answer :
Given: AB is the common chord of two intersecting circles (O, r) and (O′, r′).
To Prove: Centres of both circles lie on the perpendicular bisector of chord AB, i.e., AB is
bisected at right angle by OO′.
Construction: Join AO, BO, AO′ and BO′.

Proof: In ∆AOO′ and ∆BOO′
AO = OB [Radii of the circle (O, r]
AO′ = BO′ [Radii of the circle (O′, r′)]
OO′ = OO′ [Common]
So, ∆AOO′ ≅∆BOO′ [by SSS congruency]
Hence, ∠AOO′ = ∠BOO′ [by CPCT]
Now in ∆AOC and ∆BOC,
∠AOC = ∠BOC [∠AOO′ = ∠BOO′]
AO = BO [Radii of the circle (O, r)]
OC = OC [Common]
So, ∆AOC ≅∆BOC [by SAS congruency]
Hence, AC = BC and ∠ACO = ∠BCO ………….(i) [by CPCT]
⇒ ∠ACO + ∠BCO = 1800……….(ii) [Linear pair]
⇒ ∠ACO = ∠BCO = 900 [From equation 1 and 2]
Hence, OO’ lies on the perpendicular bisector of AB.

