Class 10 - Mathematics
Triangles - Exercise 6.4

Top Block 1
Exercise 6.4
Question : 1:Let Δ ABC ~ Δ DEF and their areas be, respectively, 64 cm2 and 121 cm2. If EF = 15.4 cm, find BC.
Answer :
Given, Δ ABC ~ Δ DEF, therefore
ar(ABC)/ar (DEF) = AB2 /DE2 = BC2 /EF2 = AC2 /DF2
Given, ar(ABC) = 64 cm2, ar(DEF) = 64 cm2, EF = 15.4 cm
⇒ ar(ABC)/ar (DEF) = BC2 /EF2
⇒ 64/121 = BC2 /(15.4)2
Take square root on both sides, we get
⇒ 8/11 = BC/15.4
⇒ BC = (15.4 * 8)/11
⇒ BC = 1.4 * 8
⇒ BC = 11.2 cm
Question : 2:Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2 CD,
find the ratio of the areas of triangles AOB and COD.
Answer :
Given, AB || DC
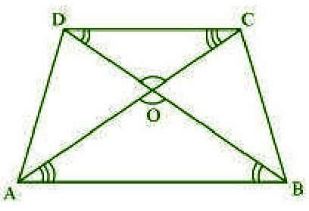
In Δ AOB and Δ COD,
∠ AOB = ∠ COD [Vertically opposite angles]
∠ OAB = ∠ OCD [Alternate angles]
∠ OBA = ∠ ODC [Alternate angles]
So, Δ AOB ~ Δ COD [AAA similarity]
Therefore, ar(AOB)/ar (COD) = AB2 /CD2
⇒ ar(AOB)/ar (COD) = (2CD)2/CD2 [Since AB = 2CD]
⇒ ar(AOB)/ar (COD) = 4CD2/CD2
⇒ ar(AOB)/ar (COD) = 4/1
⇒ ar(AOB) : ar (COD) = 4 : 1
Question : 3:In Fig. 6.44, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)/ar (DBC) = AO/DO.
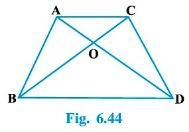
Mddle block 1
Answer :
Let AP, DM are the perpendiculars on BC.
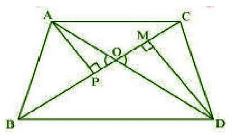
Area of triangle = (1/2) * base * perpendicular
ar(ABC)/ar (DBC) = {(1/2) * BC * AP}/{(1/2) * BC * DM} ……..1
In Δ APO and Δ DMO,
∠ APO = ∠ DMO [Each 90]
∠ AOP = ∠ DOM [Vertically opposite angles]
So, Δ APO ~ Δ DMO [AA similarity]
⇒ ar(ABC)/ar (DBC) = AO/DO [From equation 1]
Question : 4 : If the areas of two similar triangles are equal, prove that they are congruent.
Answer :
Let Δ ABC ~ Δ DEF, therefore,
ar(ABC)/ar (DEF) = AB2 /DE2 = BC2 /EF2 = AC2 /DF2 …….1
Given that ar(ABC) = ar (DEF)
⇒ ar(ABC)/ar (DEF) = 1
From equation 1, we have
AB2 /DE2 = BC2 /EF2 = AC2 /DF2 = 1
⇒ AB = DE, BC = EF and AC = DF
So, Δ ABC ≡ Δ DEF [SSS congruency theorem]
Question : 5:D, E and F are respectively the mid-points of sides AB, BC and CA of Δ ABC. Find the ratio of the areas of Δ DEF and Δ ABC.
Answer :

Therefore, DE || AC and DE = AC/2
In Δ BED and Δ BCA,
∠ BED = ∠ BCA [Corresponding angles]
∠ BDE = ∠ BAC [Corresponding angles]
So, Δ BED ~ Δ BCA [AA similarity]
ar(Δ BED)/ar (Δ BCA) = DE2 /AC2
⇒ ar(Δ BED)/ar (Δ BCA) = (AC/2)2 /AC2
⇒ ar(Δ BED)/ar (Δ BCA) = (1/4)/1
⇒ ar(Δ BED)/ar (Δ BCA) = 1/4
⇒ ar(Δ BED) =ar (Δ BCA)/4
Let ar(Δ BCA) = x
So, ar(Δ BED) = x/4
Similarly, ar(Δ CEF) = x/4 and ar(Δ ADF) = x/4
Now, ar(Δ DEF) = ar(Δ ABC) – ar(Δ BED) – ar(Δ CEF) – ar(Δ ADF)
⇒ ar(Δ DEF) = x – x/4 – x/4 – x/4
⇒ ar(Δ DEF) = x – 3x/4
⇒ ar(Δ DEF) = x/4
⇒ ar(Δ DEF) = ar(Δ ABC)/4 [Since ar(Δ ABC) = x]
⇒ ar(Δ DEF)/ar(Δ ABC) = 1/4
⇒ ar(Δ DEF) : ar(Δ ABC) = 1 : 4
Question : 6:Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Answer :

Let AD and PS are the medians of triangle.
Given, Δ ABC ~ Δ PQR
⇒ AB/PQ = BC/QR = AC/PR …………1
and ∠ A =∠ P, ∠ B = ∠ Q, ∠ C = ∠ R …..2
AD and PS are the medians of the triangle.
Therefore, BD = DC = BC/2 and QS = SR = QR/2
From the equation 1, we have
AB/PQ = BD/QS = AC/PR …………1
In Δ ABD and Δ PQS,
∠ B =∠ Q [From equation 2]
AB/PQ = BD/QS [From equation 3]
So, Δ ABD ~ Δ PQS
Therefore, AB/PQ = BD/QS = AD/PS …………4
and ar(ΔABC)/ar (ΔPQR) = AB2 /PQ2 = BC2 /QR2 = AC2 /PR2
From equation 1 and 4, we get
AB/PQ = BC/QR = AC/PR = AD/PS
Hence, ar(Δ ABC)/ar (Δ PQR) = AD2 /PS2
Question : 7:Prove that the area of an equilateral triangle described on one side of a square is equal to half the area of the
equilateral triangle described on one of its diagonals.
Answer :

Therefore, diagonal = a√2 units
The triangles form on side and diagonal are Δ ABE and Δ DBF respectively.
The length of each side of triangle ABE = a units
and the length of each side of triangle DBF = a√2 units
Both the triangles are equilateral and each angle of both the triangles are 60.
Therefore, by AAA similarity,
Δ ABE ~ Δ DBF
Now using the area theorem, we get
ar(Δ ABE)/ar (Δ DBF) = (a/a√2)2
= a2/2a2
= 1/2
⇒ ar(Δ ABE)/ar (Δ DBF) = 1/2
Tick the correct answer and justify:
Question : 8:ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the areas of triangles ABC and BDE is
(A) 2 : 1 (B) 1 : 2 (C) 4 : 1 (D) 1 : 4
Answer :
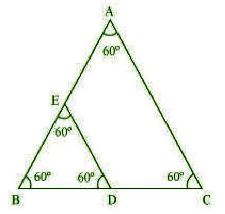
Therefore, by AAA similarity,
Δ BCA ~ Δ BDE
Therefore, the side of Δ BDE = x/2
Now, ar(Δ ABC)/ar (Δ BDE) = (x/x/2)2
= x2/(x2/4)
= 4/1
⇒ ar(Δ ABE) : ar (Δ BDE) = 4 : 1
Hence, option (C) is the correct answer.
Question : 9:Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio
(A) 2 : 3 (B) 4 : 9 (C) 81 : 16 (D) 16 : 81
Answer :
We know that the ration of similar triangles is equal to the ratio of square of their
corresponding sides.
Therefore, the ratio of areas of two triangles = (4/9)2 = 16/81 = 16 : 81
Hence, the option (D) is the correct answer.

